Welcome to the 15th Carnival of Mathematics. While the quantity is low during these doldrum months, I think the quality of this episode is excellent. The contents also speak to the question du Jore of whether the CoM should be bifurcated into separate teaching and research Carnival? We actually have a research contribution that is explainable in elementary terms and has everyday application – if you eat McNuggets everyday (not recommended)!Â
But before that, because I surely will be asked (again), let me point out I-am-only-related-by-name-to-the-late-famous-Professor-John-G-Kemeny. My favorite anecdote about him is when he was appointed President of Dartmouth he requested to continue teaching. The Board thought that was beneath the position. So he asked what they would say if he requested time to play golf. He got to teach.
My father-in-law, Bill Curnin, is a retired professor, and pointed out a Brief History of Mathematics, which he has used in the classroom since 1948. While there I noted the 1954 poem A Song … Against Mathematicians which begins:
Of all the lunatic professions which are practised on this earth
Mathematics is the craziest, and has been from its birth.
I thought to enlarge upon this in poet laureate style:
I must read and study math again, where tensor algebras Lie,
And all I need is a Kronecker delta, an epsilon and pi;
I immediately realized I wasn’t up to the poetry nor math – so you’ll have to bear with my limericks.

Chapter 1: 3599..
Taking pairs from the number nine closet,
And appending to 35, I’ll posit,
When factoring’s done,
Some 6*10N plus one,
Is a divsor. The results’ all composite!
In Teaching Factoring – Should we?, Jonathan (aka jd2718) kicks off a series of 4 articles on rationales for what we should teach our children. In the second article published today, I ban FOIL, he explains how his school handles polynomials to make factoring easier. The next article will be on the trinomial factoring technique, breaking the middle. Jonathan says, “It’s not uncommon, but many people have never seen it.” The last article will cover other sorts of factoring and anecdotes.  For reference, there are internet discussions on factoring, and/or you can just do communal factoring on your PC.
Chapter 2: A tale of five gentlemen

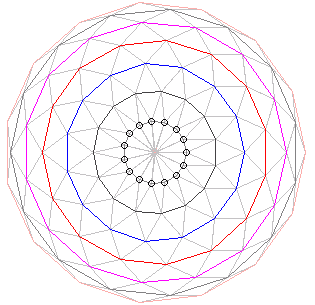
Henricus Hubertus van Aubel (1830-1906), was a Dutch mathematician who proved a pretty theorem on quadrilaterals. A. Gutierrez has a javascript conventional proof, accompanied by Chopin, online. Wesley Cowans of FOXMATHS! did one better. His proof of van Aubel’s theorem uses complex numbers instead of standard geometric techniques.
 This problem has an interesting history. It is related to a theorem attributed to Napoleon, often called the most rediscovered theorem in mathematics. Â
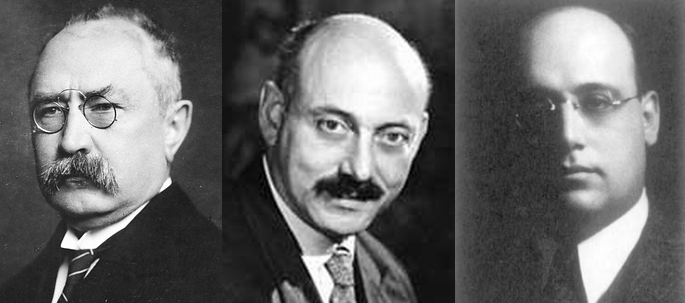 A generalization of both theorems is attributed to three men, Karel Petr, Bernhard Newmann, and Jesse Douglas (proof and interactive gizmo by Alex Bogomolny).  Petr (1868-1950) was a high-powered Czech math professor before, during, and after two World Wars – with many students-turned-refugees. Newmann has a history with Napoleon’s Theorem explained in an Australian interview entitled Napoleon, my Father and I. Douglas, co-winner of the first Fields Medal, working independently (pdf) at the same time as Newmann (1939-1941) had an incredibly prolific year in 1939. He is also a fellow Bronxite.  Of course, as Stephen Gray points out in his 2002 article Generalizing the Petr-Douglas-Neumann Theorem on n-gons (pdf), Petr has precedence, publishing in 1908! Â
A generalization of both theorems is attributed to three men, Karel Petr, Bernhard Newmann, and Jesse Douglas (proof and interactive gizmo by Alex Bogomolny).  Petr (1868-1950) was a high-powered Czech math professor before, during, and after two World Wars – with many students-turned-refugees. Newmann has a history with Napoleon’s Theorem explained in an Australian interview entitled Napoleon, my Father and I. Douglas, co-winner of the first Fields Medal, working independently (pdf) at the same time as Newmann (1939-1941) had an incredibly prolific year in 1939. He is also a fellow Bronxite.  Of course, as Stephen Gray points out in his 2002 article Generalizing the Petr-Douglas-Neumann Theorem on n-gons (pdf), Petr has precedence, publishing in 1908! Â
Chapter 3: Pay me now or and pay me laterÂ

 John Armstrong, the Unapologetic Mathematician, tackles a solution to an actual, real-world data analysis problem, CEO Compensation and its relationship to corporate profits. What relationship? John has been in an Ivory Tower too long. Read Dilbert. (just kidding – actually a thoughtful article)
John Armstrong, the Unapologetic Mathematician, tackles a solution to an actual, real-world data analysis problem, CEO Compensation and its relationship to corporate profits. What relationship? John has been in an Ivory Tower too long. Read Dilbert. (just kidding – actually a thoughtful article)
Â
Â
Â
Chapter 4:Â Study now or and study later

Dave Marain at MathNotations sent in How Recursion is Tested on the SATs and much more… Designed for middle- and high schoolers, this detailed investigation for the classroom explores the ideas of recursive-defined sequences using an SAT-type problem as a springboard.
That’s my son Alan studying at the blackboard. He should be ready for SATs in about 15 years. I wonder if they will have changed much, viz., no portable AI-bots in the exam room?
Â
Chapter 5: 263
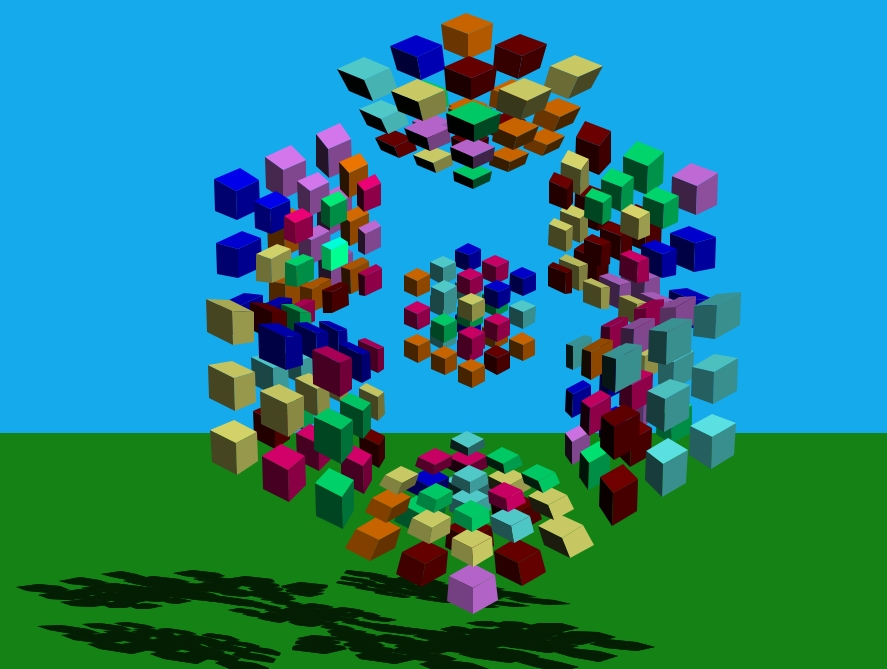 Julie Rehmeyer reports some news about advances in solving Rubik’s Cube in Cracking the Cube in MathTrek. Northeastern professor Gene Cooperman and grad student Dan Kunkle have discovered a way, using high-powered computation, to reduce the maximum number of moves to solve the puzzle by 1, to 26. However, there is room left for improvement, as it is believed that 20 moves may be the minimum. If that’s too simple, here is an online 4D Magic Cube to play.
Julie Rehmeyer reports some news about advances in solving Rubik’s Cube in Cracking the Cube in MathTrek. Northeastern professor Gene Cooperman and grad student Dan Kunkle have discovered a way, using high-powered computation, to reduce the maximum number of moves to solve the puzzle by 1, to 26. However, there is room left for improvement, as it is believed that 20 moves may be the minimum. If that’s too simple, here is an online 4D Magic Cube to play.
Julie also writes an interesting article on mathematically modeling word acquisition in Calculating the Word Spurt.
Â
Chapter 6: 43 McNuggets to don’t go
A student of Shalit’s named Xu,
Discovered some math that is new,
There are Frobenius things,
Taken from co-finite strings,
Constructed so they exponentially grew.
Here is the fresh research article, The Noncommutative Frobenius Problem is Solved!, from professor Jeffrey Shalit at Recursivity. It is well explained, and the tie-in to McNuggets is delicious.
Chapter 7: For Smarties Only, NOT!
Secret Blogging Seminar is a group blog by 8 recent and future Berkeley mathematics Ph.D.’s. Sound intimidating? Not always. Take, for example, Scott Carnahan’s piece p-adic fields for beginners. You could learn something. Then there is Noah Snyder’s The Minkowski Bound. Not frivolous!
The following rating system I devised, analogous to the movie rating system (G, PG, PG-13, R, NC-17), should help you identify appropriate articles:-)
- γ = Graduate Level
- π Γ = Post-Graduate
- Ï€ Γ – XIII = 13-year old Post-Graduate
- Ï = Rudimentary
- ν Χ – XVII= Nearly Comatose 17-year old
Epilogue: Off to the movies
That’s where I’m going. But you should stay and exercize your brain here (thanks Alvaro Fernandez & Praveen). Oh, I wanted to congratulate Sherry Gong, from Exeter, N.H., who earned a gold medal and tied for first place at the 2007 China Mathematical Olympiad for Girls, which was held in Wuhan, China, from August 11-16.










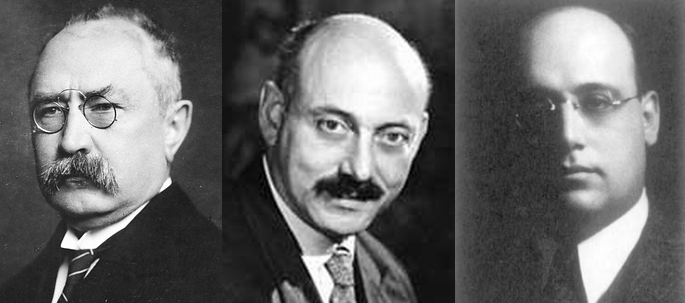 A generalization of both theorems is attributed to three men, Karel Petr, Bernhard Newmann, and Jesse Douglas (
A generalization of both theorems is attributed to three men, Karel Petr, Bernhard Newmann, and Jesse Douglas (
 John Armstrong, the
John Armstrong, the 
 Julie Rehmeyer reports some news about advances in solving Rubik’s Cube in
Julie Rehmeyer reports some news about advances in solving Rubik’s Cube in